Check out the math & physics courses that I mentioned (many of which are free!) and support this channel by going to https://brilliant.org/Sabine/ where you can create your Brilliant account. The first 200 will get 20% off the annual premium subscription. In the diagram at 4 minutes 30 seconds, the labels for h_1 and h_2 are mixed up. Sorry about that! Subscribe to my weekly science newsletter: https://sabinehossenfelder.com/ Everything I am talking about here is standard material of undergrad physics textbooks. My personal favorite is good old Goldstein https://en.wikipedia.org/wiki/Classic… On variational principles more specifically I quite like this textbook: https://link.springer.com/book/10.100… You can support me on Patreon: / sabine 0:00 Intro 0:45 Optimization 1:35 Shortest Path 3:20 Least Time 5:36 Least Action 10:27 Quantum Mechanics 11:53 Sponsor Message.
Category: mathematics – Page 56

Identifying the Neural Bases of Math Competence Based on Structural and Functional Properties of the Human Brain
It is well known that the human brain is a complex system that comprises not only individual brain regions but also distributed neural networks. The human brain is efficiently organized by integrating information across various brain regions to minimize the cost of information processing while maximizing the overall efficiency of the brain networks. Modern neuroimaging techniques allow us to identify distinct local cortical regions and investigate large-scale neural networks underlying math competence both structurally and functionally. To gain insights into the neural bases of math competence, this review aims to find answers based on structural and functional properties of the human brain in both typical and atypical populations of children and adults. Specifically, for atypical populations, we will focus on individuals with math learning deficits. Math learning deficits are neurodevelopmental disorders that impair an individual’s ability to learn and perform math-related tasks. Dyscalculia is a specific type of math learning deficit that affects the development of arithmetical skills and other basic numerical skills (Kuhl, Sobotta, Legascreen Consortium, & Skeide, 2021; Kucian et al., 2014; Rykhlevskaia, Uddin, Kondos, & Menon, 2009). When reviewing findings from atypical population, we will focus on individuals with math learning deficits including those with dyscalculia.
As math competence encompasses many different skills, for studies involving adults, this review will selectively examine the neural bases of relatively complex math skills, such as evaluation of mathematical statements (e.g., “Any equilateral triangle can be divided into two right triangles”; Amalric & Dehaene, 2016, 2019). For studies involving children, we will also include fundamental math abilities such as arithmetic skills that are commensurate with the math skills young children master. However, basic number comprehension and number comparison skills are outside the scope of this review. Moreover, we will consider whether neural markers associated with math competence are unique to math or may be reflective of academic achievement and cognitive abilities more generally.
Frontiers: Most mathematical cognition research has focused on understanding normal adult function and child development as well as mildly and moderately impaired mathematical skill
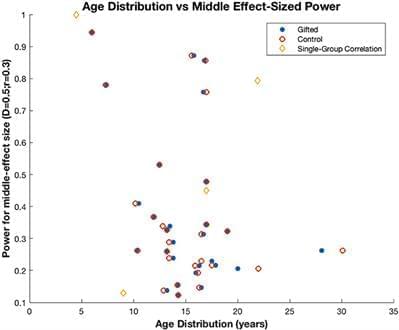
Often labeled developmental dyscalculia and/or mathematical learning disability. In contrast, much less research is available on cognitive and neural correlates of gifted/excellent mathematical knowledge in adults and children. In order to facilitate further inquiry into this area, here we review 40 available studies, which examine the cognitive and neural basis of gifted mathematics. Studies associated a large number of cognitive factors with gifted mathematics, with spatial processing and working memory being the most frequently identified contributors. However, the current literature suffers from low statistical power, which most probably contributes to variability across findings. Other major shortcomings include failing to establish domain and stimulus specificity of findings, suggesting causation without sufficient evidence and the frequent use of invalid backward inference in neuro-imaging studies. Future studies must increase statistical power and neuro-imaging studies must rely on supporting behavioral data when interpreting findings. Studies should investigate the factors shown to correlate with math giftedness in a more specific manner and determine exactly how individual factors may contribute to gifted math ability.
A disproportionately large amount of scientific advancement throughout history has occurred due to cognitively gifted individuals. However, we know surprisingly little about the cognitive structure supporting gifted mathematics. The current understanding is that human mathematical ability builds on an extensive network of cognitive skills and mathematics-specific knowledge, which are supported by motivational factors (Ansari, 2008; Beilock, 2008; Fias et al., 2013; Szűcs et al., 2014; Szűcs, 2016). To date, most psychological and neuroscience studies have examined potentially important factors only in children and adults with normal mathematics as well as in children with poor mathematical abilities (e.g., in children with mathematical learning disability or developmental dyscalculia). In contrast, those with high levels of mathematical giftedness received relatively little attention.

AI now beats humans at basic tasks — new benchmarks are needed, says major report
Artificial intelligence (AI) systems, such as the chatbot ChatGPT, have become so advanced that they now very nearly match or exceed human performance in tasks including reading comprehension, image classification and competition-level mathematics, according to a new report (see ‘Speedy advances’). Rapid progress in the development of these systems also means that many common benchmarks and tests for assessing them are quickly becoming obsolete.
These are just a few of the top-line findings from the Artificial Intelligence Index Report 2024, which was published on 15 April by the Institute for Human-Centered Artificial Intelligence at Stanford University in California. The report charts the meteoric progress in machine-learning systems over the past decade.
In particular, the report says, new ways of assessing AI — for example, evaluating their performance on complex tasks, such as abstraction and reasoning — are more and more necessary. “A decade ago, benchmarks would serve the community for 5–10 years” whereas now they often become irrelevant in just a few years, says Nestor Maslej, a social scientist at Stanford and editor-in-chief of the AI Index. “The pace of gain has been startlingly rapid.”
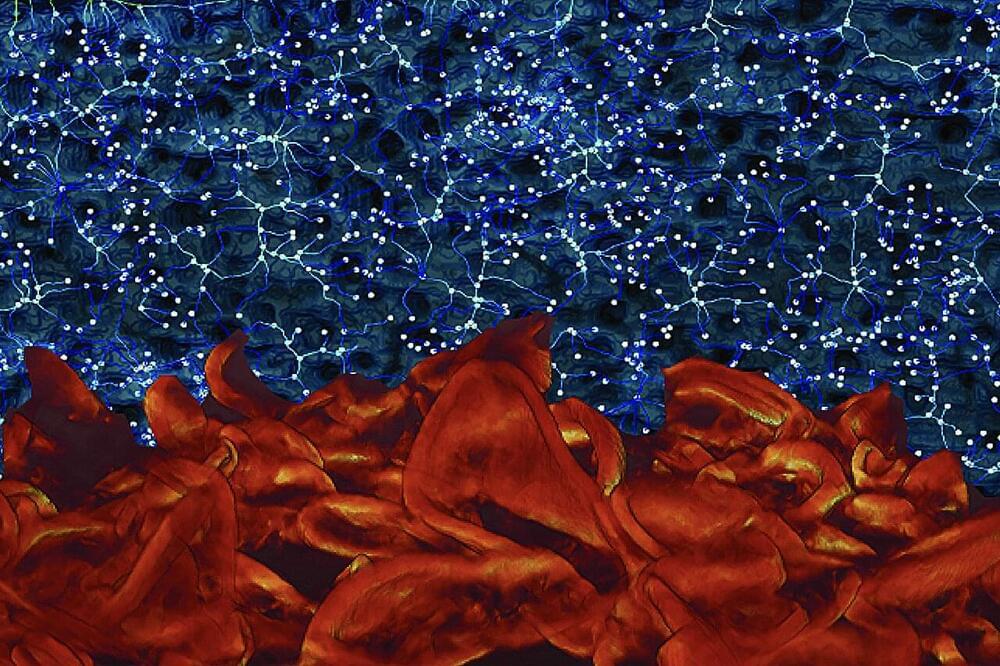
Nothing is everything: How hidden emptiness can define the usefulness of filtration materials
Voids, or empty spaces, exist within matter at all scales, from the astronomical to the microscopic. In a new study, researchers used high-powered microscopy and mathematical theory to unveil nanoscale voids in three dimensions. This advancement is poised to improve the performance of many materials used in the home and in the chemical, energy and medical industries—particularly in the area of filtration.
Magnification of common filters used in the home shows that, while they look like a solid piece of material with uniform holes, they are actually composed of millions of randomly oriented tiny voids that allow small particles to pass through. In some industrial applications, like water and solvent filtration, paper-thin membranes make up the barriers that separate fluids and particles.
“The materials science community has been aware of these randomly oriented nanoscale voids within filter membranes for a while,” said Falon Kalutantirige, a University of Illinois Urbana-Champaign graduate student.
Warp Drives: New Simulations
Learn more from a science course on Brilliant! First 30 days are free and 20% off the annual premium subscription when you use our link ➜ https://brilliant.org/sabine.
Hyperjumps, wormholes, and warp drives sound like science fiction, but they’re actually based on real science! Though I believe out of the three, warp drives are the most plausible. The math seems to agree. Today I want to tell you about a new way of analysing and visualizing warp drives.
Code: https://github.com/pbbp0904/WarpFactory.
🤓 Check out my new quiz app ➜ http://quizwithit.com/
💌 Support me on Donatebox ➜ https://donorbox.org/swtg.
📝 Transcripts and written news on Substack ➜ https://sciencewtg.substack.com/
👉 Transcript with links to references on Patreon ➜ / sabine.
📩 Free weekly science newsletter ➜ https://sabinehossenfelder.com/newsle…
👂 Audio only podcast ➜ https://open.spotify.com/show/0MkNfXl…
🔗 Join this channel to get access to perks ➜
/ @sabinehossenfelder.
🖼️ On instagram ➜ / sciencewtg.
#science #sciencenews #warpdrives #physics

Unlocking AI’s Black Box: New Formula Explains How They Detect Relevant Patterns
A UC San Diego team has uncovered a method to decipher neural networks’ learning process, using a statistical formula to clarify how features are learned, a breakthrough that promises more understandable and efficient AI systems. Credit: SciTechDaily.com.
Neural networks have been powering breakthroughs in artificial intelligence, including the large language models that are now being used in a wide range of applications, from finance, to human resources to healthcare. But these networks remain a black box whose inner workings engineers and scientists struggle to understand. Now, a team led by data and computer scientists at the University of California San Diego has given neural networks the equivalent of an X-ray to uncover how they actually learn.
The researchers found that a formula used in statistical analysis provides a streamlined mathematical description of how neural networks, such as GPT-2, a precursor to ChatGPT, learn relevant patterns in data, known as features. This formula also explains how neural networks use these relevant patterns to make predictions.

This Math Problem Stumped Scientists for Almost a Century — Two Mathematicians Have Finally Solved It
We’ve all been there: staring at a math test with a problem that seems impossible to solve. What if finding the solution to a problem took almost a century? For mathematicians who dabble in Ramsey theory, this is very much the case. In fact, little progress had been made in solving Ramsey problems since the 1930s.
Now, University of California San Diego researchers Jacques Verstraete and Sam Mattheus have found the answer to r(4,t), a longstanding Ramsey problem that has perplexed the math world for decades.

The multiverse could be much, much bigger than we ever imagined
A new way of interpreting the elusive mathematics of quantum mechanics could fundamentally change our understanding of reality.
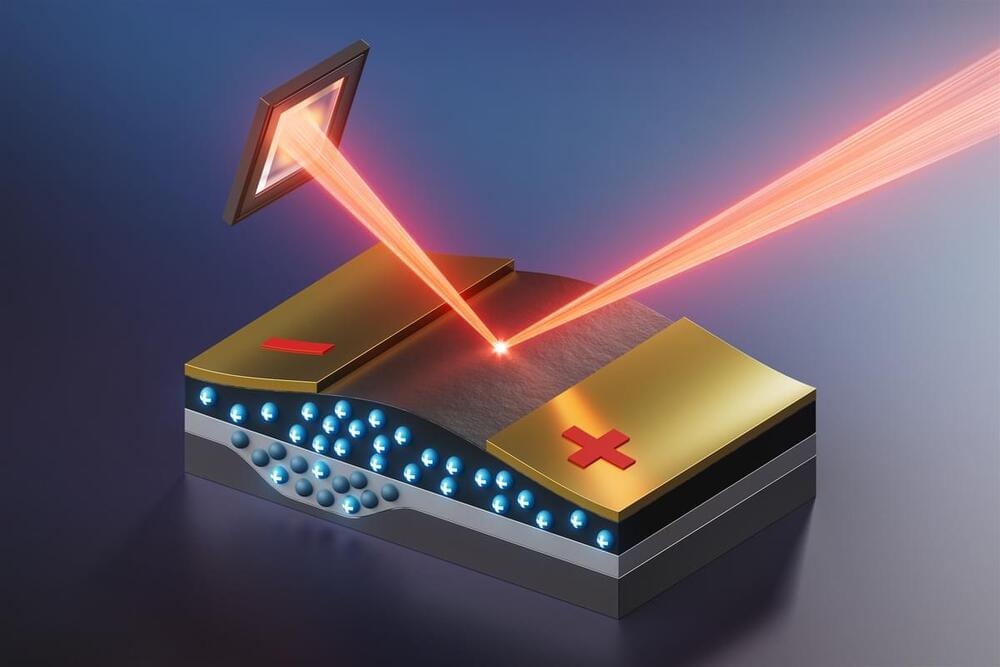
Advanced imaging techniques on a semiconductor material reveal ‘surprising’ hidden activity
“We found to our great surprise that this substrate is very much active, jiving and responding in completely surprising ways as the film switches from an insulator to a metal and back when the electrical pulses arrive,” Gopalan said. “This is like watching the tail wagging the dog, which stumped us for a long while. This surprising and previously overlooked observation completely changes how we need to view this technology.”
To understand these findings, the theory and simulation effort — led by Long-Qing Chen, Hamer Professor of Materials Science and Engineering, professor of engineering science and mechanics and of mathematics at Penn State — developed a theoretical framework to explain the entire process of the film and the substrate bulging instead of shrinking. When their model incorporated naturally occurring missing oxygen atoms in this material of two types, charged and uncharged, the experimental results could be satisfactorily explained.
“These neutral oxygen vacancies hold a charge of two electrons, which they can release when the material switches from an insulator to a metal,” Gopalan said. “The oxygen vacancy left behind is now charged and the crystal swells up, leading to the observed surprising bulging in the device. This response can also happen in the substrate. All of these physical processes are beautifully captured in the phase-field theory and modeling performed in this work for the first time by the postdoc Yin Shi in Prof. Chen’s group.”