Aug 10, 2023
Quantum material exhibits ‘non-local’ behavior that mimics brain function
Posted by Dan Breeden in categories: computing, information science, mathematics, neuroscience, quantum physics
We often believe computers are more efficient than humans. After all, computers can complete a complex math equation in a moment and can also recall the name of that one actor we keep forgetting. However, human brains can process complicated layers of information quickly, accurately, and with almost no energy input: recognizing a face after only seeing it once or instantly knowing the difference between a mountain and the ocean.
These simple human tasks require enormous processing and energy input from computers, and even then, with varying degrees of accuracy.
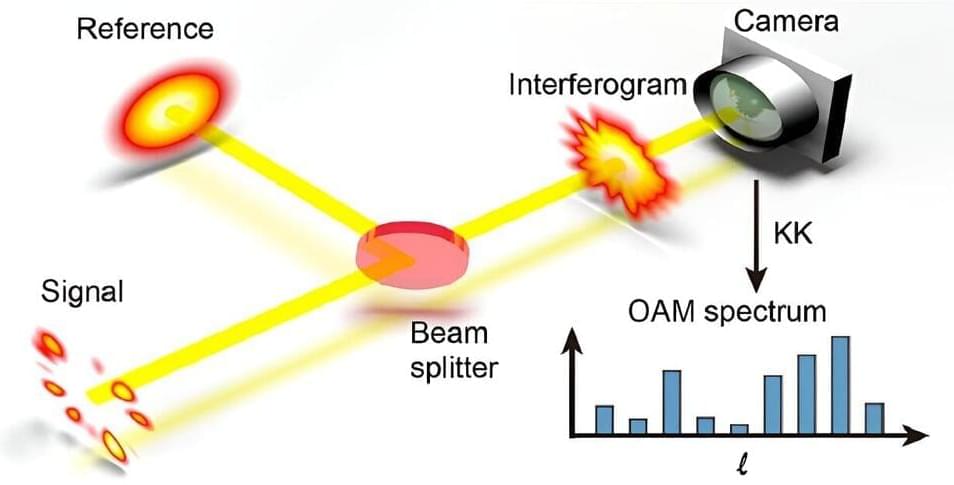
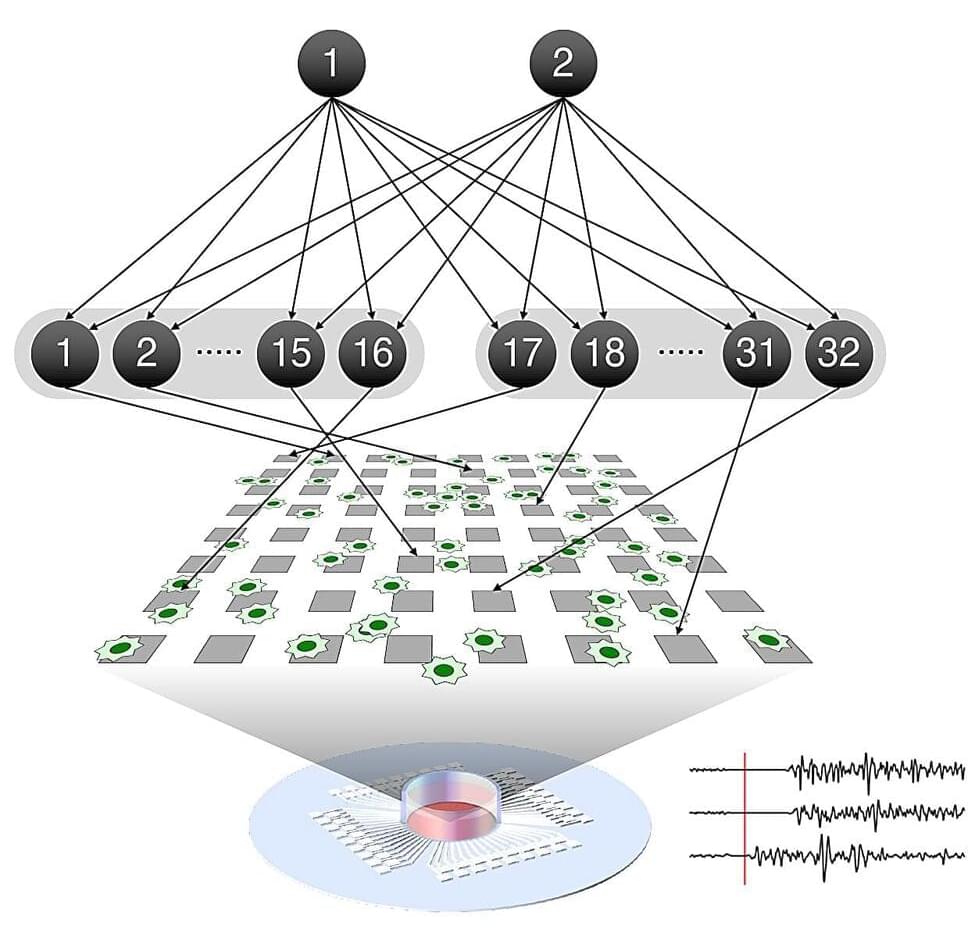
Creating brain-like computers with minimal energy requirements would revolutionize nearly every aspect of modern life. Quantum Materials for Energy Efficient Neuromorphic Computing (Q-MEEN-C)—a nationwide consortium led by the University of California San Diego—has been at the forefront of this research.